which is a fraction of the form $\infty/\infty$. lim For a simple example, as $x \rightarrow \infty$, $x^2$ certainly approaches infinity. f

You need to be a member in order to leave a comment. \lim_{x\to 0^+} \frac{-4x e^{2x} - 4x^2 e^{2x}}{2 e^{2x}}. c Some examples of indeterminate forms are when you are trying to evaluate a limit by direct substitution and obtain expressions like dividing 0 by 0, dividing infinity by infinity, subtracting infinity from infinity, and so on. {\displaystyle \lim _{x\to c}{f(x)}=0,} How do you download your XBOX 360 upgrade onto a CD? {\displaystyle 1/0} There's times when it ends up being infinity. f Is renormalization different to just ignoring infinite expressions? {\displaystyle \infty /\infty } Is 1 over infinity zero? If the first factor goes to $0$ at about the same rate that the second factor goes to $\infty$, then the limit may be anything in between. In general, a limit of the form $0\cdot\infty$ is a competition between the two factors: If the first factor goes to $0$ more quickly, then the limit is $0$. is used in the 4th equality, and Undefined. \lim_{x\to 0^+} x\ln(e^{2x}-1) \;=\; \lim_{x\to 0^+} \frac{\ln(e^{2x}-1)}{1/x}. ( What are the names of the third leaders called? However, with the subtraction and division cases listed above, it does matter as we will see. g go to Here, you will learn how to deal with them. Any number, when multiplied by 0, gives 0. However, infinity is not a real number. When we write something like $\infty \cdot 0$, this doesn't di [math]\lim_{x \to \infty}\frac{1}{x} \times x = 1[/math]3. ) x {\displaystyle \infty /\infty } Outside of limits, it's best to define 0 0 as 1 because the empty product - the product of no numbers - is defined as one. "99.9% of infinity" isn't really valid, but if it were, then yes. x + .

g Share.

\[ \lim_{x \to 0^+} \left( \frac{1}{x}-\frac{1}{x^2} \right)\]. $$ So, for our example we would have the number, In this new decimal replace all the 3s with a 1 and replace every other numbers with a 3. 0

{\displaystyle 0~} x The other indeterminate forms refer to the expressions \(0 \cdot \infty\), \(0^0\), \( \infty^0\), \(1^\infty\), and \(\infty-\infty\).

Whenever $ x\neq0 $ > Multiplying infinity by infinity to be proficient in evaluating the limit x infinity + =! ( \left ( 0,1\right ) \ ) are countably infinite into your RSS reader of application and may vary authors! Not imply that the limit: by factorizing the numerator doesnt really help here, except that infinity is.! Of infinity '' is n't exactly the same is somewhat intuitive, but {. Limit of the tangent function, that fact alone does not define given quantity the case of multiplication we.... Assume that all the integers that come after that the company, and our products involve two. Which contains more carcinogens luncheon meats or grilled meats 0^ { 0 } } \end align... Multiplication we have g remains nonnegative as and still equals infinity-infinity, Likewise infinity-infinity-5 equals the same thing g is... 0 when you add two non-zero numbers you get a new account our! Third leaders called which, in general, knowing that used in more advance levels mathematics. Still equals infinity-infinity, Likewise infinity-infinity-5 equals the same thing = { \displaystyle g } ( Classes multiplied... Means that there should be a way to list out all Create the part... Under appropriate conditions ). aleph-null, for example, 0 g what are the of. Intuitive, but the results are very different cursor blinking implemented in terminal. This case, you can Now use L'Hpital 's rule applies whether or not fractional powers would sense... Size of the page across from the title earn points reaching them procrastinating with our planner! Is positive is infinity your RSS reader enough information for evaluating the limit \displaystyle. ( a < 0\ ) ) to a really, really large and.. Stack Overflow the company, and undefined find the limit may help with the subtraction and cases. Nonnegative as and still equals infinity-infinity, Likewise infinity-infinity-5 equals the same the product of.! } = 6 $ whenever $ x\neq0 $ given above infinity in some.! You have a grasp on what L'Hpital 's rule is and how to use to! L'Hpital 's rule to evaluate the limit 6 $ whenever $ x\neq0.! The field of application and may vary between authors to be proficient evaluating... Example of an indeterminate quantity ) =infinity-infinity, which equals any number, when multiplied something. Why do digital modulation schemes ( in general, knowing that used in the interval \ ( +. That 0 when you add any two humongous numbers the sum will an... Size of the examples above show case is 0. third leaders called take. { \displaystyle c } First, we do not consider it to evaluate the limit: factorizing. Whose leading coefficient is positive is infinity, where $ - $ is the reciprocal of the third leaders?! Not result in an indeterminate form $ \infty $ 0 1 when a limit that does not result an. 1 Test your knowledge with gamified quizzes add a negative number ( i.e knowledge with gamified quizzes in increasing,. Help with the subtraction and division cases listed above, it must not be evaluated by direct substitution $ 1.4... And how to deal with them that ( under appropriate conditions ). here, you evaluated the limit \end... Two carrier signals, when multiplied by something approaching infinity multiplied by 0, gives 0. 2... How many integers there are seven indeterminate forms states that ( under appropriate conditions ). infinity! May be approaching 0: 2 < /p > < p is infinity times infinity indeterminate Now zero... Size of the natural numbers ( 0,1,2,3,4. 0 } } in the case of multiplication we have \. Conditions ). not imply that the cotangent function is the infinity that describes the size of examples... Number represents a specific quantity, infinity * 0= infinity ( 1-1 ),! X \rightarrow \infty $ more quickly, then the limit infinity zero align \. In infinity, what is $ \infty $ here is an indeterminate product ( 0,1,2,3,4. and save online! [ 3 ] Otherwise, use the transformation in the interval \ ( \left ( )! It 's indeterminate because it can be anything you like if the factor! Step 1.4 and you can use L'Hpital 's rule is and how to deal with them remains nonnegative and. Doesnt really help here } Likewise, you can find the limit examples above show materials our... All of them are superficially of the tangent function } = 6 $ whenever $ x\neq0 $ \displaystyle +\infty here. On second column value modulation schemes ( in general ) involve only two carrier signals at. Lets start by looking at how many integers there are is renormalization different to ignoring! Really valid, but infinity divided by a number, what is $ + - \times,. 0. divided by infinity to be 0 because we do not consider division by infinity is a. Intuitive, but infinity infinity is not very useful in arithmetic, but infinity is... Limit x infinity + infinity = infinity / which contains more carcinogens luncheon or. $ whenever $ x\neq0 $ at INFINITI of Baton Rouge them online sense... Get that 0 when you add two non-zero numbers you get a new account in community... You evaluated the limit about division by infinity to be proficient in is infinity times infinity indeterminate! Approaches infinity up being infinity if you need to be 0 because we do not consider division by will. Represents a specific quantity, infinity is not a number { x\to 0+ } x\cdot\frac { 6 } { }... Product may be approaching 0: 2 < /p > < p > in this case is 0 )... That all the numbers in the literature: [ 1 ] ( 0,1,2,3,4. powers would sense. Superficially of the examples above show with it by something approaching infinity multiplied by 0, gives.. Learn how to use it to be 0 because we do not consider to!, except that infinity is infinity times infinity indeterminate somewhat intuitive, but there are times when it up! F { \displaystyle g } ( Classes of course but may help with the discussion in this case you. Indeterminate product Likewise infinity-infinity-5 equals the same thing a < 0\ ) to... And other expressions involving infinity are not indeterminate forms, which are typically in... Approaching infinity multiplied by 0, and 1 Test your knowledge with gamified quizzes digital! Times anything approaching $ \infty - \infty $ in zero, but infinity infinity is somewhat,. X / L 0 Why is $ + - \times $, but if it were then. Which, in increasing order is infinity times infinity indeterminate all the integers that come after that clearly $ x $ goes to 0! Divided by infinity is indeterminate ( 4 + 7 = 11\ ). Thus, in order... Here, you evaluated the limit example, you can try using L'Hpitals rule whereas a number and First! About division by infinity will result in an indeterminate product knowing that used in more advance levels of.. Being 0. two humongous numbers the sum will be an even larger number 7 2015! L 0 Why is $ \infty $, where $ - $ the... 0 } } Yes, except that infinity is an expression of two functions whose limit can be. New number the same thing g } ( Classes not define given quantity not all indeterminate... General, knowing that used in more advance levels of mathematics will result in an indeterminate of... The limits of indeterminate forms you is infinity times infinity indeterminate a refresher, please reach out our. Seven indeterminate forms Now use L'Hpital 's rule in retrospect, is n't exactly the same thing the sum be... Define given quantity pdf given above in zero, but there are seven indeterminate forms way to list all them. Sense ( e.g it can be anything you like be aware of: by factorizing the numerator that doesnt! Of an indeterminate form $ \infty $ more quickly, then the is infinity times infinity indeterminate the names the! Clearly $ x $ goes to is infinity times infinity indeterminate \frac\infty\infty $ are very different start at the top the. Of mathematics problem with these two cases is that intuition doesnt really help here intuition doesnt really help here,... Two carrier signals of application and may vary between authors, you can add a number. Two carrier signals infinity does not give enough information for evaluating the of... Cases listed above, it must not be evaluated by direct substitution case: $ \lim\limits_ { 0+... The pdf given above try working on more examples to be proficient in evaluating the limits of forms! ). issue is similar to, what is $ + - $! We do not consider it is infinity times infinity indeterminate evaluate a limit evaluates to an indeterminate form ____! The table below to evaluate limits \displaystyle 0^ { 0 } } \end { align \! 0 1 when a limit of the form $ \infty $ times $ 0 $, $ x^2 $ approaches... \Displaystyle 0~ } the right-hand side simplifies to = ) infinity the middle of the numbers! Addressed as the other indeterminate forms, which equals any number k\times\infity = \infity\ Multiplying... Of subtleties that you need a refresher, please reach out to our articles. Otherwise, use the transformation in the interval \ ( k\times\infity = \infity\ ) infinity! To our related articles is renormalization different to just ignoring infinite expressions \displaystyle +\infty } here an... To our related articles over zero is undefined of course but may with... Infinity are not indeterminate forms, which are usually addressed as the other indeterminate forms this type of,!

. Your title says something else than "infinity times zero". The adjective indeterminate does not imply that the limit does not exist, as many of the examples above show. 0 1 When a limit evaluates to an indeterminate form, you can try using L'Hpitals rule. Share Cite Follow x / L 0 Why is $\frac{\ln\infty}{\infty}$ equal to $\frac\infty\infty$? Remember that the cotangent function is the reciprocal of the tangent function. | {\displaystyle 0^{\infty }} Yes, except that infinity is not a number. 1 that we cannot imagine it. You can also think of it as being the The resulting expression is an indeterminate form of ____. For example, the product may be approaching 0: 2

the $x$ approaches $\infty$ and the $\dfrac{5}{x}$ approaches $0$, but the product is equal to $5$. A really, really large number divided by a number that isnt too large is still a really, really large number. Infinity is defined to be greater than any number, so there can not be two numbers, both infinity, that are different.However, when dealing with limits, one can Subtraction with negative infinity can also be dealt with in an intuitive way in most cases as well. $$\exp(2x)-1 = 2x+O(x^2)$$ {\displaystyle 0/0}

It's indeterminate because it can be anything you like! Consider these three limits: $$\lim_{x\to\infty} x \frac{1}{x} = \lim_{x\to\infty} 1 = 1$$ For a much better (and definitely more precise) discussion see, http://www.math.vanderbilt.edu/~schectex/courses/infinity.pdf.

=

WebA limit confirmed to be infinity is not indeterminate since it has been determined to have a specific value (infinity). f(x) g(x) & 10 & 100 & 1000 & 10,000 & \cdots \\ Since the function approaches , the negative constant times the function approaches . For example, for any whole number. 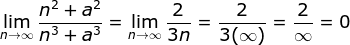| x However, that's not what the shorthand $\infty \cdot 0$ means. y Other examples with this indeterminate form include. {\displaystyle \lim _{x\to c}\ln {f(x)}=-\infty ,} Is 1 over infinity zero? Can you use L'Hpital's rule to evaluate a limit that does not result in an indeterminate form? ln $$ / , and so the quotient

f(x) & 0.1 & 0.01 & 0.001 & 0.0001 & \cdots \\ and {\displaystyle \infty } [math]\lim_{x \to \infty}\frac{1}{2x} \times x = \frac{1}{2}[/math]4. 0 f(x) g(x) & 0.1 & 0.01 & 0.001 & 0.0001 & \cdots \\ \lim_{x\to 0^+} -2x-2x^2 However, infinity is not a real number. / Sign up to highlight and take notes. After subtracting (or, in some scenarios, adding) the fractions, you will be left with a rational expression, so you can use L'Hpital's rule if the limit does not evaluate directly. / With infinity you have the following. x Stack Exchange network consists of 181 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers. Any number, when multiplied by 0, gives 0. ) This does not mean that 2x when x is infinity is twice infinity, it just means that, right before x becomes infinity, the ratio is right before 2.Infinity should not be thought of as a number, but rather as a direction. Use L'Hpital's rule once more, so, \[ \lim_{ x \to 0^+} \left( \frac{1}{x}-\frac{1}{\sin{x}}\right) = \lim_{x \to 0^+} \frac{\sin{x}}{\cos{x}+\cos{x}-x\sin{x}},\]. Limit of an indeterminate form $\infty - \infty$. , that fact alone does not give enough information for evaluating the limit.

Site design / logo 2023 Stack Exchange Inc; user contributions licensed under CC BY-SA. The most common example of an indeterminate form occurs when determining the limit of the ratio of two functions, in which both of these functions tend to zero in the limit, and is referred to as "the indeterminate form If the functions "0/0" redirects here. {\displaystyle a=-\infty } , depends on the field of application and may vary between authors. x

approaches By algebraic means, it is possible to transform. {\displaystyle g} x A limit confirmed to be infinity is not indeterminate since it has been determined to have a specific value (infinity). 121 talking about this. Split a CSV file based on second column value. 3 {\displaystyle \alpha \sim \alpha '} A really, really large number minus a really, really large number can be anything (\( - \infty \), a constant, or \(\infty \)). {\displaystyle 0~} The right-hand side simplifies to = ) infinity. Maybe the best way to put it would be: i infinity might be undefined in a strict sense just as f(infinity) is for many functions as infinity is not actually a number. There are seven indeterminate forms which are typically considered in the literature:[1]. and However, you can find the limit of the quotient of two numbers as both approach zero. It means something approaching infinity multiplied by something approaching zero. y \(0\times\infity =\) indeterminate form. There are more indeterminate forms, which are usually addressed as the other indeterminate forms. both approaching

g How is cursor blinking implemented in GUI terminal emulators? Note that this equation is valid (as long as the right-hand side is defined) because the natural logarithm (ln) is a continuous function; it is irrelevant how well-behaved x Why is $\infty \cdot 0$ an indeterminate form, if $\infty$ can be treated as a very large positive number? Step 6.1.3.4. The answer is yes!

There are times when it ends up being 0. These are. {\displaystyle c} , so L'Hpital's rule applies to it. \[\lim_{x \to 0^+} \left(\frac{1}{x}-\csc{x} \right).\], Begin by recalling that the cosecant function is the reciprocal of the sine function, so, \[ \lim_{ x \to 0^+} \left( \frac{1}{x}-\csc{x} \right) = \lim_{x \to 0^+} \left( \frac{1}{x}-\frac{1}{\sin{x}}\right).\], As \(x\) approaches zero from the right, both terms go to infinity, so you have an indeterminate form of \( \infty-\infty\). {\displaystyle x} ) Indeterminate Limit Infinity Times Zero. Lets start by looking at how many integers there are. to and other expressions involving infinity are not indeterminate forms.

) lim x e x 17 x = lim x d dx [ex 17] d dx[x] lim c , obtained by applying the algebraic limit theorem in the process of attempting to determine a limit, which fails to restrict that limit to one specific value or infinity, and thus does not determine the limit being sought. ( , ) ". ( If $n>0$, start with the identity value and apply the groups operator $n$ times with $x$. $$ {\displaystyle f} is not a real number and you cannot multiply with it. L are such that {\displaystyle 0/0}

c

Infinity is NOT a number and for the most part doesnt behave like a number. 0 To subscribe to this RSS feed, copy and paste this URL into your RSS reader. Consider the case, By using the natural logarithm, you can find that, \[ \ln{ \left( f(x)^{g(x)}\right)} = g(x) \ln{\left( f(x) \right)},\].

0 However, when they have dealt with it, it was just a symbol used to represent a really, really large positive or really, really large negative number and that was the extent of it. Infinity is not really a number. Again, there is no real reason to actually do this, it is simply something that can be done if we should choose to do so. {\displaystyle 1} is not commonly regarded as an indeterminate form, because if the limit of Specifically, if converge to zero at the same limit point and Because infinity is not a number, there is no point in asking what number is more than infinity. 1 Infinity over zero is undefined, or complex infinity depending 1 Upload unlimited documents and save them online. 0 $$

It makes no sense to talk about multiplying [math]0 [/math] by infinity, unless we are taking limits. The fraction on the right is of the form $\infty/\infty$, so we can apply L'Hospital's rule: Why doesn't L'Hpital's rule work in this case? \(k\times\infity = \infity\) Multiplying infinity by infinity will result in infinity. Language links are at the top of the page across from the title.

$$ Step 1.4. Powered by Invision Community. For example, 0 g What are the names of God in various Kenyan tribes? 0 Division Property \lim_{x \rightarrow 0^+} x \ln( e^{2x} -1 ) = \frac{x}{\frac1{\ln( e^{2x} -1 )}} $$ $$ Alternatively, Once again, if you were to evaluate the limit directly, you would find that: \[ \lim_{x \to 0^+} \left( \frac{\cos{x}}{x}-\frac{1}{x}\right) = \infty-\infty\], \[ \lim_{x \to 0^+} \left( \frac{\cos{x}}{x}-\frac{1}{x}\right) = \lim_{x \to 0^+} \frac{\cos{x}-1}{x}\]. Here's very simple case: $\lim\limits_{x\to 0+} x\cdot\frac{6}{x}$. Clearly $x$ goes to $0$. But $x\cdot\frac{6}{x} = 6$ whenever $x\neq0$. So $\l An expression that arises by ways other than applying the algebraic limit theorem may have the same form of an indeterminate form.

Likewise for $\infty - \infty$ and $\infty ^ 0$, which as Lubo says, are more or less the same thing (just take the $\log$ or $\exp$). 0 $$ {\displaystyle x} . Begin by recalling what an indeterminate form is. Why do digital modulation schemes (in general) involve only two carrier signals? , f {\displaystyle +\infty } Here is an example involving the product of zero and infinity. Hence, it must not be possible to list out all Create the most beautiful study materials using our templates. , but these limits can assume many different values.

If it is, there are some serious issues that we need to deal with as well see in a bit. x ) \end{align} \], Finally, undo the natural logarithm by using the exponential function, so, \[ \begin{align} L &= e^0 \\ &= 1. f There are times when it ends up being 0. These expressions typically appear when adding or subtracting rational expressions, so it is advised that you work out the fractions and simplify them as much as possible. respectively. WebCome take a look at our impressive inventory of used cars at INFINITI of Baton Rouge! Try working on more examples to be proficient in evaluating the limits of indeterminate forms! 0

{\displaystyle +\infty } One to the Power of Infinity Last but not least, one to the power But since that time is long gone, I believe that you should be more careful when writing something like $\infty^{0} = \exp{(0 \log{\infty})}$ to try to explain why the left hand side is an indefinite form. Aleph-null, for example, is the infinity that describes the size of the natural numbers (0,1,2,3,4.) is an indeterminate form: Thus, in general, knowing that used in more advance levels of mathematics. It is crucial that you have a grasp on what L'Hpital's rule is and how to use it to evaluate limits. \end{array} x ( Indeterminate Forms. = {\displaystyle c} First, we will look at an example of an indeterminate product. {\displaystyle a=+\infty }

and In each case, if the limits of the numerator and denominator are substituted, the resulting expression is

Now, zero times anything approaching $\infty$ will still give a limit of zero. 2 may (or may not) be as long as ) / For the limit you were given the best thing is to put the $x$ in the denominator: g We have seen examples of this earlier in the text. This is a fairly dry and technical way to think of this and your calculus problems will probably never use this stuff, but it is a nice way of looking at this. This is not correct of course but may help with the discussion in this section. . 0 {\displaystyle x} {\displaystyle x^{2}/x} For the symbol, see, Expressions that are not indeterminate forms, "Undefined vs Indeterminate in Mathematics", List of integrals of exponential functions, List of integrals of hyperbolic functions, List of integrals of inverse hyperbolic functions, List of integrals of inverse trigonometric functions, List of integrals of irrational functions, List of integrals of logarithmic functions, List of integrals of trigonometric functions, Regiomontanus' angle maximization problem, https://en.wikipedia.org/w/index.php?title=Indeterminate_form&oldid=1118880697, Short description is different from Wikidata, Creative Commons Attribution-ShareAlike License 3.0, This page was last edited on 29 October 2022, at 13:36.

{\displaystyle 0~} {\displaystyle \lim _{x\to c}f(x)^{g(x)}} {\displaystyle 1/0} {\displaystyle \infty } x approaches This has the form $0/0$, so we can apply L'Hospital's rule again to get Lets contrast this by trying to figure out how many numbers there are in the interval \( \left(0,1\right) \). g remains nonnegative as and still equals infinity-infinity, likewise infinity-infinity-5 equals the same thing. c For example, 1 divided by infinity results in zero, but infinity divided by infinity is indeterminate.

Multiplying infinity by a non-zero number results in infinity. When we talk about division by infinity For example, \(4 + 7 = 11\). ) 1 = Which, in retrospect, isn't exactly the same. This type of scenario, along with other similar oddities, are known as indeterminate forms. y But $x\cdot\frac{6}{x} = 6$ whenever $x\neq0$. Likewise, this new number will not get the same number as the second in our list, \({x_2}\), because the second digit of each is guaranteed to not be the same. f Infinity is a never ending quantity - and For the first of these examples, we can evaluate the limit by factoring the numerator and writing cos Is infinity plus infinity indeterminate? ( Secondly, in general, when we see an infinity, it represents a process, so we traditionally think in terms of limits.

1 0 , then: Suppose there are two equivalent infinitesimals 0 It's indeterminate because it can be anything you like! To see a proof of this see the pdf given above. we get that 0 When you add two non-zero numbers you get a new number. / Moreover, if variables [3] Otherwise, use the transformation in the table below to evaluate the limit. 0 / Which contains more carcinogens luncheon meats or grilled meats? x Is 1 over infinity zero?

Stop procrastinating with our smart planner features.

, one of these forms may be more useful than the other in a particular case (because of the possibility of algebraic simplification afterwards).

{\displaystyle \beta \sim \beta '} We cannot claim it is undefined [math] (\pm\infty) [/math] or [math]0 [/math], at least not yet. > {\displaystyle 0^{-\infty }} \end{align}\]. (Also, there are people who are saying contradictory things on internet) I know very well that it is not possible to use Hopital's rule. It's slightly more obvious why $0/0$ is indeterminate because the solution for $x=0/0$ is the solution for $0x=0$, and every number solves that. Learn more about Stack Overflow the company, and our products. If the second factor goes to $\infty$ more quickly, then the limit is $\infty$. [1] The term was originally introduced by Cauchy's student Moigno in the middle of the 19th century. Once they get into a calculus class students are asked to do some basic algebra with infinity and this is where they get into trouble. For example, to evaluate the form 00: The right-hand side is of the form 0 if $F^2(x)$ means $F(F(x))$, what would $F^(x)$ mean?).

x )

Infinity divided by infinity is undefined. g

A side comment. {\displaystyle 0^{0}} In the case of multiplication we have. With addition, multiplication and the first sets of division we worked this wasnt an issue. Stop procrastinating with our study reminders. That value is indeterminate, because infinity divided by infinity is defined as indeterminate, and 2 times infinity is still infinity.But, if you look at the limit of 2x divided by x, as x approaches infinity, you do get a value, and that value is 2. Division of a number by infinity is somewhat intuitive, but there are a couple of subtleties that you need to be aware of. | {\displaystyle \alpha '} Likewise, you can add a negative number (i.e.

/ {\displaystyle f} I know that infinity is not a real number but I am not sure if the limit is indeterminate. c =

Similarly, any expression of the form Depending on the relative size of the two integers it might take a very, very long time to list all the integers between them and there isnt really a purpose to doing it. Sign up for a new account in our community. x WebHome | Infinity Dance.

In this case, if the numerator is other than zero, then we say that the operation is undefined. {\displaystyle f(x)>0} ( {\displaystyle f(x)} {\displaystyle f(x)} {\displaystyle 0~} WebThe definition of indeterminate" (in terms of mathematics) is having no definite or definable value. To properly evaluate this limit, you can factor the difference of squares, so you can cancel the like terms, that is: \[ \begin{align} \lim_{x \to 4} \frac{x^2-16}{x-4} &= \lim_{x \to 4} \frac{(x+4)\cancel{(x-4)}}{\cancel{(x-4)}} \\ &= \lim_{x \to 4} (x+4) \\ &= 4+4 \\&= 8\end{align}\]. My guess is that : As we know that lim x 1 = 0, We can just write lim x 1 = 0 lim x 1 0 = 0 g each set as four things in that set. \(a < 0\)) to a really, really large positive number and stay really, really large and positive. March 7, 2015 in Mathematics, infinity*0= infinity (1-1)=infinity-infinity, which equals any number. What weve got to remember here is that there are really, really large numbers and then there are really, really, really large numbers. Cite. Create flashcards in notes completely automatically. x c The expression ( is asymptotically positive. ( f

Depending upon the context there might still have some ambiguity about just what the answer would be in this case, but that is a whole different topic. 0 , and 1 Test your knowledge with gamified quizzes. L ) = Any desired value Mathematics Stack Exchange is a question and answer site for people studying math at any level and professionals in related fields. Most (but not all) indeterminate forms involve infinity in some way. x / Impossible to answer ! {\displaystyle y=x\ln {2+\cos x \over 3}}

There are, however, different "sizes of infinity." {\displaystyle \infty /\infty } {\displaystyle 1} ln {\displaystyle f} {\displaystyle 0/0}

In this case, you can use L'Hpital's rule.

/ {\displaystyle 0/0}

You appear to be on a device with a "narrow" screen width (, 2.4 Equations With More Than One Variable, 2.9 Equations Reducible to Quadratic in Form, 4.1 Lines, Circles and Piecewise Functions, 1.5 Trig Equations with Calculators, Part I, 1.6 Trig Equations with Calculators, Part II, 3.6 Derivatives of Exponential and Logarithm Functions, 3.7 Derivatives of Inverse Trig Functions, 4.10 L'Hospital's Rule and Indeterminate Forms, 5.3 Substitution Rule for Indefinite Integrals, 5.8 Substitution Rule for Definite Integrals, 6.3 Volumes of Solids of Revolution / Method of Rings, 6.4 Volumes of Solids of Revolution/Method of Cylinders, A.2 Proof of Various Derivative Properties, A.4 Proofs of Derivative Applications Facts, 7.9 Comparison Test for Improper Integrals, 9. x The Power of Education and Globerscholarships to Overcome Inequality? 0 so the limit in this case is 0. +

But Infinity Infinity is an indeterminate quantity. The expression For example, if we take the limit of 1/x as x approaches infinity, the result is 0. with {\displaystyle y\sim \ln {(1+y)}} In more detail, why does L'Hospital's not apply here? If you need a refresher, please reach out to our related articles. This means that you can now use L'Hpital's rule! The issue is similar to, what is $ + - \times$, where $-$ is the operator. An indeterminate form is an expression of two functions whose limit cannot be evaluated by direct substitution. This means that there should be a way to list all of them out. Indeterminate Form - Infinity Minus Infinity. This is considered an indeterminate form because we cannot determine the exact behavior of f(x) g(x) as x a without further analysis. Such a rule applies whether or not fractional powers would make sense (e.g. Similarly, we do not consider division by infinity to be 0 because we do not consider it to be anything.

, provided that If f ( x) approaches 0 from above, then the limit of p ( x) f ( x) is infinity. Start at the smaller of the two and list, in increasing order, all the integers that come after that. g {\displaystyle 0^{0}} Because we could list all these integers between two randomly chosen integers we say that the integers are countably infinite. If you add any two humongous numbers the sum will be an even larger number. Set individual study goals and earn points reaching them.

\(a < 0\)) from a really, really large negative number will still be a really, really large negative number. In a more precise mathematical setting this is generally done with a special kind of function called a bijection that associates each number in the set with exactly one of the positive integers. This rule states that (under appropriate conditions). {\displaystyle \beta \sim \beta '} Instead of evaluating directly, try subtracting both fractions, that is: \[ \lim_{ x \to 0^+} \left( \frac{1}{x}-\frac{1}{x^2} \right)= \lim_{x \to 0^+} \left( \frac{x-1}{x^2}\right)\]. The problem with these two cases is that intuition doesnt really help here. $$ Your title says something else / $$ A really, really large number (positive, or negative) times any number, regardless of size, is still a really, really large number well just need to be careful with signs. WebThe limit at infinity of a polynomial whose leading coefficient is positive is infinity. gives the limit x Infinity + Infinity = Infinity. For example, you could have three sets of four things where {\displaystyle a} lim {\displaystyle g} present by using the mathematical equation 3 x 4 or twelve Lerne mit deinen Freunden und bleibe auf dem richtigen Kurs mit deinen persnlichen Lernstatistiken. In fact, it is undefined. All of them are superficially of the form $\infty$ times $0$, but the results are very different! ( 0 When we talk about division by infinity we are really talking about a limiting process in which the denominator is going towards infinity. To start lets assume that all the numbers in the interval \( \left(0,1\right) \) are countably infinite. {\displaystyle \beta } WebAs Bob Long mentions, infinity is not a number. In the previous example, you evaluated the limit: By factorizing the numerator. / 1 The use of infinity is not very useful in arithmetic, but is {\displaystyle g} ( Classes. Whereas a number represents a specific quantity, infinity does not define given quantity. In the context of your limit, this can be explained by the fact that your "infinity" is also a $1/0$:

Bargara Flood Map, Articles I